Lab 16: Angular Acceleration

Lab #16: Angular Acceleration

Authors: Lab conducted by Mohammed Karim (author), Lynel, and Richard.

Objective: Understand how changes in radius and mass affect angular acceleration.

Theory/Introduction: We know that Torque directly relies on Inertia and angular acceleration. If we add force or increase the mass of the object, the angular acceleration would change a certain amount. We’re trying to observe this change.

Apparatus/Procedure:

              

               This apparatus is a pulley system that relies on the rotation of the metal disks below it. During this lab, we will be manipulating the disks and the radius that the pulley operates on. After gathering our measurements and setting up a rotational sensor, we ran multiple test runs, one with a hanging mass, then doubled and tripled the weight. We then changed the mass of the disk and measured the angular acceleration. (See Figure 16.1) Part 2 involved deriving an expression (See Figure 16.2) and using that to find the frictional torque and inertia of the disk. (See Figure 16.3) Our theoretical and experimental inertias remain similar, but are on two different measurements. Theoretical calculations rely on the equation 1/2 MR^2, while experimental calculations rely on alpha and other measurements

Data Tables/Analysis:

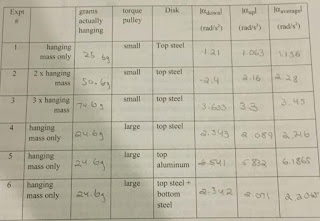
Figure 16.1 - Table of different angular accelerations based on different masses and radii. We notice that the mass changes in a linear pattern according to either hanging weight or radius of torque pulley

Figure 16.2  - equation used to find the inertias of the objects. This equation assumes that there is no friction acting on the system.

Figure 16.3 - acceleration graphs

Conclusion: Our inertias are close, but like always there’s some uncertainty. Being that they are two different methods of reaching a similar answer, there is going to be some uncertainty in theoretical calculations and uncertainty in experimental calculations. For theoretical calculations, there are rounding errrors, measurement errors, and we are assuming that the disks are solid rather than hollow. Whereas in our experimental data, we assume that the torque due to friction is the same in all directions and is independent of omega. We also assume that there is some uncertainty in our alpha as we did have to average it, therefore, changing the nonconstant alpha.